Besides being one of the most important relationships in all of quantum physics, Heisenberg's Uncertainty Principle is something that a lot of disgruntled high schoolers will complain about having to learn in grade 12 Chemistry. Unfortunately, a lot of people will toss the principle to the side because they won't take the time to understand it. If said student does decide to tackle the principle at a deep level, they too will often end up dismissing it because it just sounds absurd.
Like most of quantum mechanics, I will concede that the principle does indeed sound bizarre, especially if you try to relate to our macroscopic world. In this post, I'll try to take a different angle and attempt to explain it mathematically (to some degree). For some, I think this may be more helpful.
Now, there are a couple of important things to take note of here:
For starters, there absolutely is experimental backing to this idea. This is not just something we pulled out of a hat and have blindly accepted. The idea has been tested time and time again.
Perhaps more importantly however (depending on your view point), this is not just some relationship, vaguely modelling reality, that we've determined solely through observation. The math behind it absolutely legitimate and sound.
Here is the mathematical inequality that describes the uncertainty principle:
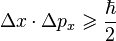
Where delta X (the triangle with the X) is the uncertainty in position and delta Px (the triangle with the Px) is the uncertainty in momentum. The dot between them means multiplication. On the right hand side, you have "h bar" which represents Planck's constant divided by 2 Pi. It is a fundamental constant that is very important at extremely small scales.
Now, you might notice a couple of things that immediately pop out. For starters, what happens if my uncertainty in position is 0 (in other words, if I am absolutely certain about the particle's position)? Well, let's sub in 0 for Delta X. On the left side we'll have…just 0, since 0 times any number is going to be 0. This gives us an inequality of:
Like most of quantum mechanics, I will concede that the principle does indeed sound bizarre, especially if you try to relate to our macroscopic world. In this post, I'll try to take a different angle and attempt to explain it mathematically (to some degree). For some, I think this may be more helpful.
Now, there are a couple of important things to take note of here:
For starters, there absolutely is experimental backing to this idea. This is not just something we pulled out of a hat and have blindly accepted. The idea has been tested time and time again.
Perhaps more importantly however (depending on your view point), this is not just some relationship, vaguely modelling reality, that we've determined solely through observation. The math behind it absolutely legitimate and sound.
What is the Uncertainty Principle?
Here is the mathematical inequality that describes the uncertainty principle:
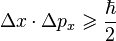
Where delta X (the triangle with the X) is the uncertainty in position and delta Px (the triangle with the Px) is the uncertainty in momentum. The dot between them means multiplication. On the right hand side, you have "h bar" which represents Planck's constant divided by 2 Pi. It is a fundamental constant that is very important at extremely small scales.
Implications
Reading this out, what this relationship says is that there is a minimum product for the uncertainties in position and momentum. The product of the two must always be greater than or equal to h-bar divided by 2 (but NEVER less than).Now, you might notice a couple of things that immediately pop out. For starters, what happens if my uncertainty in position is 0 (in other words, if I am absolutely certain about the particle's position)? Well, let's sub in 0 for Delta X. On the left side we'll have…just 0, since 0 times any number is going to be 0. This gives us an inequality of:
0 > h-bar/2
(Sorry, I couldn't get the fancy letters :)
Uh-oh! That is not a valid inequality! While h-bar over 2 is an extremely small number (something like 5.0 x 10^-35…That's 0.000000000000000000000000000000000005…or something like that), it is STILL bigger than 0, making this inequality untrue.
So, now we have determined that, according to the inequality, we can NEVER be absolutely certain about a particle's position or a particle's momentum. Some uncertainty must exist between the two.
Now, there is another implication that is visible to us all upon a closer look:
As the certainty in one of the variables (either position or momentum) increases, the certainty in the other decreases.
Now why is this? Well, let's look at the inequality again! If the certainty in a particle's position, for example, were to increase, this means that its uncertainty decreases. This results in an ever shrinking Delta X. I can get really close to certain with the particle's position, but remember that I can't be absolutely certain.
But wait! We can't make Delta X too small! That's because we have to maintain the inequality. If Delta X gets super small, that will make the left side of the inequality smaller because a number times a very small number, equals a very small number. Remember that the left side NEEDS to be bigger than the right side, no matter how small Delta X gets (and it can definitely get really, really close to zero). So, how do we combat this? By increasing the momentum (Delta Px)!
But we don't have to do anything! Nature does it for us! That's right: to maintain this inequality, as my certainty in the position increases, my uncertainty in momentum will increase.
This is done to ensure that the left side maintains large enough to satisfy the inequality. And keep in mind, all of this is backed my experiment. Position and momentum are like dials on a machine that are intrinsically linked together: Tweaking the dial on one will result in a change in the other (technically speaking, it is an inverse relationship).
So, there you have it! Explained above are two of the main implications of Heisenberg's Uncertainty Principle with the mathematical foundations behind them! Perhaps next week, I can make a post on commuters and the non-commutative properties of quantum mechanics.
(SNEAK PEAK: 2 x 3 does not equal 3 x 2 in quantum mechanics…)
Comments
Post a Comment